by Poul Anderson
as seen in "Astounding Science Fiction" November 1956.
|
A personal experience with this article -
P.S. I have asked Poul's widow for permission to web publish this piece, but she wishes more "bread" than I am willing to offer. She died in 2018. |
We, it happens, use length, time, and mass as the fundamental units against which all else is measured. It's obvious that these are the only proper fundamentals . . . or is it? Planck's Constant, which appears to be a true universal, is the quantum of action, a hybrid of time and energy ...
Let's call him Przewalski, just to get away from the monotonously Anglo-Saxon names of science-fiction heroes. It does seem improbable that as small a minority of humankind as those of North European ancestry will forever be the leaders of Terrestrial civilization. Przewalski was an eminent young physicist, head of the scientific mission to Vega Five.
Explorers from the Solar System had turned out to be the first in this neck of the galaxy equipped with a hyperspatial drive; but man was by no means the only civilized race. In many respects the Vegans were ahead of us, and an exchange of knowledge was indicated.
Landing at the planet's largest city, the humans donned their airsuits, glare filters, spore repulsors, and a dozen other items required to keep them alive on this "terrestroid" world. The Vegans crawled hospitably forth to meet them, wagging tails in the most ceremonious manner, and led the way to specially prepared quarters. Banquets, receptions, the conferring of honorary degrees, and speeches on the "hands-across-space" theme took only a week-though to be sure, Vega Five has a ninety-hour day. At length Przewalski found himself conferring through a glass partition with his opposite number. The being's name was quite unpronounceable by any human - special voder equipment was needed for the discussion - so we will return to science-fiction tradition and call him Jennings.
"Getting down to business," said Przewalski, "the most obvious difference between the accomplishments of our two peoples is that we know more about hyperspace and you know more about radiation. You ought to, with this sun of yours! Suppose I ask you a few questions, just to start the ball rolling."
"What ball?" asked Jennings. When it had been explained, he nodded. "Oh, I see. The proper idiom is 'to strike the gong with vigor and enthusiasm.' "
Przewalski sighed, drew a deep breath, and went on: "I've heard that you have discovered a quantized structure in the photon. Could you outline the theory for me?"
"Easily. The structural unit is the guwiggl (rough approximation to a horrible noise) which is expressed in terms of glutch times thirk - Oh, dear." Jennings wrung his hands, all six of them. "Your linguists of the preceding expedition never did think to inquire about the special language of physics."
"Well, we can figure it out," said Przewalski. "Is the quwiggl a unit of energy?" Perforce, he used the Esperanto word for "energy." "That is, well, one form of energy is given by the integral . . . damn! . . . now let me see. Look, you have a certain mass, a certain amount of matter. Understand? You exert a certain force on it - a push, a pull. This accelerates it, makes it speed up. The force is equal to the mass times the acceleration, and the work done, the energy expended, is the force times the distance through which it acts. Understand ?"
"No," said Jennings unhelpfully. "Gluich is - " He went into a long rigmarole. Przewalski finally got the idea that glutch was capacitance. Jennings realized what mass and energy are, but he thought of them as functions of capacitance, action, and radiation flux.
At the end of a rather unprofitable session, Jennings gave Przewalski some books on elementary Vegan physics. Then he crawled home, shaking his ears in mild dismay that the Earthlings should have based their physics on something so utterly trivial as mass.
Przewalski settled doggedly down to read his way through. He got past the first sentence, and stopped cold. What was a huk? Looking it up, he found it to be a unit of distance. It was the length of one side of a cube of water with a capacitance of one glutchguggl. Przewalski groaned and reached for his slide rule and Rubber Handbook.
He was going to be on Vega Five a long, long time.
All of which is a roundabout introduction to a most interesting and complicated subject, the matter of units and dimensions. It is one which, on the practical side, has bedeviled us for centuries with the end not yet in view. On the theoretical side, it touches the philosophical foundations of science.
Every traveler abroad has run into the problem of conversion. If a sign-post announces it is forty-five kilometers to Paris . . . how many miles? The American or Englishman is so used to thinking in terms of his own weird measurements that he normally has to translate before kilometers and kilograms have real meaning for him. But this is a minor nuisance compared to what the technical student must go through. An electric motor puts out twenty horsepower . . . let's see, how many kilowatts does that amount to? One horsepower is 0.7457 kW, or is it the other way around? Probably as many examination questions are missed because of multiplying by the wrong conversion factor as for any other reason; humanely, most instructors only take off a few points for this mistake.
It seems grossly unfair that we must wrestle with twelve inches to the foot, five thousand two hundred eighty feet to the mile, sixteen ounces to the pound, two pints to the quart, and one hundred sixty square rods to the acre, when the non-English-speaking world has nothing more to do than multiply sensible units by some power of ten. Who's responsible?
As usual, no one person is to blame. Human history looks like a series of bumbling accidents. The metric system originated in France and was adopted during the Revolution. The Anglo-Saxon countries, including the United States, wanted nothing to do with any project nurtured by wild-haired regicides, and stayed with weights and measures going back to the Middle Ages. By the time we were able to look at it rationally, it was too late. There was too large an investment in machinery built in English units for us to scrap. Of course, machinery does wear out and can be replaced by freshly designed equipment, but skilled mechanics last somewhat longer, and they are used to thinking in inches rather than centimeters. To them a centimeter is only an intellectual concept, with no "feel." It would take many years to train our labor force into new habits, and meanwhile work would be slowed down. ("Micrometer reads 10.493 cm., now how many inches is that?") The long-range saving in time and effort would be worth the trouble, but mankind isn't noted for thinking very far ahead.
The European continent was fortunate. It had less industry in the Eighteenth Century than England, so the changeover was easier. Even so, it was not made overnight; the process was only finished two or three generations ago. Some people, like the Germans, helped matters along by slapping a special tax on everything not built or sold in the new measurements.
Vestiges of the old system linger on. I have seen them in action. It is required by law in Denmark that groceries be sold by metric units; but it happens that half a kilo is approximately one pound. So the Danes still go to the store and ask for a pound of butter, receiving 0.5 kilogram.
Ironically, the United States is officially on the metric system. An Act of Congress in the last century created legal definitions of our English units in metric terms. But that's no help in everyday life,
Let's glance at the metric system and see what it actually is. Everybody knows that the meter was defined as a fraction of the Earth's circumference and that the gram is supposed to be the mass (not weight) of one cubic centimeter of water at four degrees Centigrade, the point of maximum density. But these are not the true definitions. After all, the eighteenth-century measurements were not too precise; any physical unit is subject to change as measuring techniques improve. Strictly speaking, the meter is the distance between two parallel scratches on a metal bar kept at a controlled temperature in Sevres, France. The International Standard Kilogram is, likewise the mass of a particular material object stored in the same vaults.
Still - those scratches have a finite width. There is a certain range of error which is too great for the modern physicist, dealing as he does with quantities like one electron mass. There has been a proposal to re-define the meter in terms of the wave length of cadmium red light. A standard which cannot easily be lost, stolen, or tampered with. But then, on the other hand, maybe those theorists are right who hold that the wave lengths of all radiations are slowly changing -
The same problem arises in creating units of time - which, thank God, are the same all over the world, though divisions based on twelve are rather clumsy in a number system based on ten. We can define the second as a certain fraction of the Earth's rotation period; but this period fluctuates occasionally, a phenomenon called trepidation, and in any case is gradually increasing because of tidal drag.
From the philosopher's view point, science is a cat's cradle of interrelated phenomena, tied down to nothing except the immediate sense data of the observer. If the entire universe. including ourselves and our measuring instruments, is uniformly shrinking or expanding, we have no means of knowing it. The proposition is, in fact, devoid of empirical content.
But we have to start somewhere. As we make fresh discoveries, we must return to our basic concepts and give them fresh definitions. It seems unlikely that we will ever know just what is meant by a "centimeter," a "gram," or a "second." There will be definitions, both verbal and operational, but the full meaning, the total implication, will always escape us.
All our units are arbitrary. The circumference of the Earth, its rotation period. or the density of water can scarcely have my cosmic significance. It has been suggested that we might adopt a set of "natural" units, based on such quantities as the rest mass of the electron, the associated wave length, and the velocity of light Such systems have been worked out. But they don't represent any great gain: they are subject to the same errors of measurement, the same prospect of future revision. Nor do they simplify calculation, since other natural quantities are not neat multiples of the proposed base units.
It appears that the metric system is still our best bet. Physical scientists throughout the world have been sensible enough to adopt it, in the CGS form--the fundamental units being the centimeter, the gram, and the second. There is, however, another metric system favored by engineers, the MKS: meter, kilogram, and second. The difference is more than a question of which power of ten to multiply by; certain quantities and equations, especially in electromagnetic theory, assume different forms and dimensions because such other natural constants as the permittivity of free space have been assigned different values. Personally, I was weaned on CGS and am prejudiced in its favor but I must admit that MKS is easier to use in some branches of physics.
I am pretty sure that the English speaking peoples won't hold out forever. Eventually Americans, too, will be measuring their distances in kilometers, though no doubt the British will make exceptions for such ancient streets as the "Royal Mile" of Edinburgh. The "Royal One-point-six Kilometer" just doesn't sound right.
But when we start dealing with extraterrestrial civilizations -- oh, brother! The inhabitants of Jupiter, if any, may be able to tell us a lot about high-pressure chemistry. But look at a handbook, with its million or so entries, and imagine having to convert everything from snorks (3.98742 inches) to centimeters! The Jovians will sit back and grin, because their system is based on the number eight, the sidereal year of their planet, and the physical properties of ammonia at standard Jovian temperature and pressure.
Of course, this is only a mechanical problem, which we could turn over to computers. But suppose the aliens use an altogether different set of basic concepts?
This brings us to the meat of the present article: the question of dimensions. For those who have nor worked in physics, dimensionality is a complex topic, and even professional scientists rarely realize the full implications.
That word "dimension" has been grossly misused in science-fiction, and we had better take time to see what it really means. In workaday language, 1 dimension is a length, as when we say the dimensions of a box are 6'x6'x10'. It's clear enough that "length" of an object is arbitrary and can be measured in any direction.
We also speak of three-dimensional space. This means no more and no less than that three co-ordinates are necessary and sufficient to define a point in that space. A line is a one dimensional space: having once fixed a zero point, we need only a single number to specify any other point in the line. A sheet of paper is two dimensional: we have to draw an x and a y axis. All the above are Euclidean. But a curve may be thought of as a one-dimensional space, the surface of a sphere as a two dimensional space, and so on; these are the nonEuclidean spaces encountered by the average man.
But suppose we are investigating the physics of gases. In order to determine precisely the state of a gas, we must list a great many quantities. such as molecular weight, pressure, temperature, degree of ionization, and so on. The biologist, and still more the sociologist, must denumerate hundreds or millions of independent variables to specify a state - in these two cases, we still don't know what most of the variables are, we only know there are a lot of them. The total state of the system is a function of all these and if each of them is given a numerical value, the function gets a value, a single number which describes the state of the system.
Therefore -- any such function can be thought of as a space with dimensionality equal to the number of independent variables needed. Such a space is known to mathematical physicists as a "phase space," and can have any old number of dimensions. Thus, the phase space of system of electrons has three dimensions for each electron involved. Every point in a phase space defines a certain state of the system under consideration.
Ordinary Euclidean 3-space, such as man once imagined himself to inhabit, is merely the phase space of a single rigid body. The only thing it describes is the position of such a body. in principle, it doesn't even have to be Euclidean.
One of the non-Euclidean spacer is of particular interest, being that of a relativistic universe. We might as well be clear on one point: it is not legitimate to ray that the cosmos is a Riemannian space. What we mean is that the theoretical geometric construct of relativity is Riemannian, and that there appears to be correspondence between this "map" and the structure of physical data.
As everyone knows by now, the Einsteinian universe is four-dimensional: besides the usual x, y, and z co-ordinates, we need a fourth t coordinate to specify the time of an event. What is not so well known is the fact that this t co-ordinate does not have the same character as the others. You can transform an x axis into a y by a simple rotation, but the transformation of t involves multiplication by the velocity of light and the square root of minus one.
From all the foregoing, it should be plain that the old science-fiction theme of Invaders from Another Dimension is pure nonsense. You might as well speak of Invaders from Length. In fact, it's precisely as meaningful to speak of Invaders from Hunger.
Because actually a dimension is any measurable quantity in which we happen to be interested. You can plot the alcoholic content of beer against the temperature of fermentation every bit as readily as you can plot the position of a bullet against the time it left the gun. A dimension can be length, time, weight, electric charge, cost, birth rate, pie-eating ability--to borrow an example from L. Sprague de Camp-or anything else.
But naturally some dimensions are a trifle more fundamental than others. Pie-eating ability can be expressed as mass consumed per second, whereas it would get rather complicated if we defined mass and time in terms of pie-eating ability.
Newton made clear the distinction between mass and weight. (Though some science-fiction writers, whose heroes have no trouble picking up a thousand-ton spaceship on a small asteroid, still haven't gotten it through their heads.) Mass appeared to be a basic quantity, the mass of an object would be the same anywhere in the universe. Length seemed another such fundamental unit, since area and volume can be expressed as powers of length. And time could hardly be questioned in those days; how would they have defined time as a function of anything else?
It has been shown that three dimensions are necessary to and sufficient to describe all the quantities of physics. The three we have chosen on Earth are mass, length, and time. (I pass over the rather difficult question of temperature.) For instance, velocity is distance (length) per unit of time; acceleration is velocity per unit of time; force it mass times acceleration; energy (work) is force times distance...and so on. These dimensions behave exactly like ordinary algebraic symbols.
This point must be emphasized if we are to develop our line of argument. Let's abbreviate mass; length, and time as m, l, t respectively. Then velocity has the dimensions lt-1, acceleration lt-2, force mlt-2, energy ml2t-2, and so on. (For the benefit of those whose algebra is even rustier than mine, a negative exponent indicates division and a fractional exponent the root to be extracted.) It is worthwhile showing a case in which the dimensions of some quantity ire to be found. How about electric charge?
In the CGS (electrostatic) system, the force between two charges in free space is equal to the product of the charges divided by the square of the distance between them. If I may be permitted an equation, this is
assuming that the two charges are equal. By simple algebra, then,
or, in dimensions,
Working this out, we see that the coulomb is
In verbal language, a coulomb is the square root of a gram times the cube of the square root of a centimeter, per second -- a ghastly mess, but perfectly unambiguous.
I solemnly swear that the above are the only equations in this article.
Certain quantities are dimensionless, e.g., specific heat. This does not make them any less real, it only indicates that they are comparative. The late Sit Arthur Eddington found dimensionless quantities which were algebraic combinations of such natural constants as Planck's - one of them, for example, was the ratio of mass between the electron and proton. These Eddington numbers are independent of the units chosen; the ratio of two masses is the same whether they be expressed in grams, pounds, or Martian ziks. He then set himself the incredible task of deriving these numbers from a few simple axioms. His death cut short a work which might have changed our whole concept of the nature of the universe, of logic, and of the human mind. But that is unfortunately not relevant here.
The dimensions of some quantities depend on those chosen for others. Thus, if we wished, we could make the gravitational constant a dimensionless absolute with a value of unity, but this would require us to define either mass, length, or force differently.
This simple case illustrates a surprising and important fact which has hardly been noticed by anyone. We have to choose three fundamental units, yes, but which three we choose is, in principle at least arbitrary.
As a matter of fact, there is already one set of units which does not take mass is a starting point This is the FPS (foot-pound-second) system of the English-speaking engineer, in which the pound is not a mass but a force. Here on Earth it makes small practical difference, but out in space, in free fall, the distinction between mass, a scalar, and force, a vector, would rapidly become obvious.
But all this is pretty small potatoes when we think of the systems which extraterrestrial scientists might pick.
Mass, length, and time looked fundamental to our ancestors, and we are now stuck with them. But a native of Vega Five might attach more importance to the amount of radiation he is getting per square centimeter per second, the energy flux, than to the total amount he receives in a day. When his giant sun stands at high noon, the flux might be too great for him to venture outdoors. His physicists could well have substituted energy flux for time - though from his viewpoint, it's we who have switched things around. And come to think of it, when you travel from one place to another, it isn't the distance you're primarily interested in, it's the energy and time required to make the trip. In this sense, a round-about road, well-paved and with little traffic is shorter than a straight but overcrowded highway; an can go faster and more easily on the first route. So the Vegans might well imagine action (energy times time) to be more important than a mere distance - an attitude which would pay off when they got around to develop quantum theory. As for their basic unit... well, suppose their early scientists happened to find out more about electrostatics than mechanics. (This could have happened-in the Hellenistic era of Earth, but didn't.) They would be inclined to think of the capacitance of a body as more important than its mass. When they eventually figured out statics and dynamics, they would get the idea of mass all right, but for them it would be an auxiliary concept rather than a basic one.
Or take Hal Clement's fine novel "Mission of Gravity." You remember that his planet Mesklin was enormous, flattened out by its terrific rotational speed, with the force of gravity radically dependent on the latitude you happened to be at. In their prescientific age, the Mesklinites would have no way of realizing that mass is constant, but they would be acutely aware of the changing force on them as they traveled about. Under the high gravity of the polar regions, they could never get up much speed, but the acceleration of a falling body would be an important characteristic of any locality. And when they began to breed physicists, the rapid rotation of the planet would suggest an intensive study of spinning bodies. It would soon become clear that the weight and size of a rotating object were less useful in predicting its behavior that the angular momentum.
So let us imagine that the Mesklinites worked out a physics based on force, acceleration, and angular momentum. Let us call these dimensions f, a, and w respectively. Then let's see what their other units would become.
The accompanying table shows three systems of dimensions. The first column represents the CGS system of Earth. The exponents of mass, length, and time are shown in that order. For instance, we read from the table that torque has the dimensions ml2t-2, gram-square centimeter per second per second, the same as energy. To find the corresponding Mesklinite units, set up their f, a, and w as functions of m, 1, and t, and solve for the latter; then you can go right down the CGS column making substitutions to get the faw column. We find, then, that on Mesklin torque has the dimensions f1/2a1/2w1/2, the square root of force timer acceleration times angular momentum.
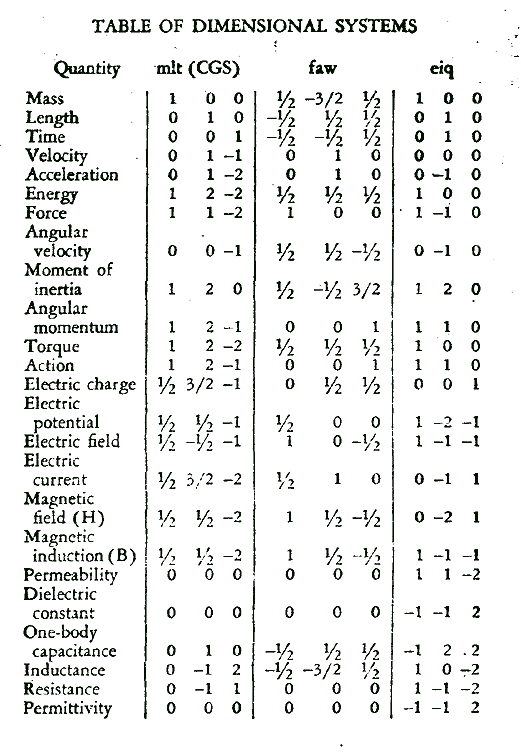
Some interesting details show up. Velocity and acceleration have the same dimension, a. A Mesklinite understands the difference between speed and the rate at which speed is acquired, but it isn't a really fundamental distinction to him. His mass, length, and time are other messy functions of f, a, and w. He would, of course, have names for such quantities, just as we speak of "coulombs" other than of m1/2l3/2t-1. Electrical resistance turns out to he dimensionless, a comparative quantity. Planck's Constant, as with us, has the dimension of angular momentum. but the Mesklinite student would see this at a glance while we must have it pointed out to us. On the other hand, - he would be slower to realize that one-body capacitance has the dimensions of length.
Earth Jupiter, Vega Five, Mesklin - in a welter of such conflicting units the scientists of an interstellar civilization would have some trouble exchanging information. Perhaps they would get together and try to work out a universal set of dimensions, corresponding to qualities they believe to be genuinely fundamental. After all, we on Earth can see that the Vegans and the Mesklinites were being arbitrary to the point of frivolity; as for us, Einstein has shown that mass, length, and time are nor universal constants either but dependent on the velocity of the observer.
Electric charge could be a good base point for this new interstellar system. Energy seems to-be quite important too. And for our third quantity, how about interval? This is given by the square root of X2 plus Y2 plus Z2 minus c2T2, where X, Y, and Z are the spatial distances between two events and T the time between them. Though X, Y, Z, and T may all be measured differently by different observers, the interval is invariant - the same for all.
The third column of the table shows an energy-interval-charge (eiq) system of dimensions. It is not converted directly from CGS, but embodies some advantageous features of MKS. You will note that mass has the dimensions which was to be expected, and velocity is dimensionless, which reflects well the fact that velocity is relative. Acceleration comer out to be i-1 and force is ei-1, energy per unit of interval. Not bad. It becomes still more attractive when we see that the eiq system is entirely free of there fractional exponents.
But there's a catch. Some quantities which are dimensionless in CGS acquire dimensions in eiq. Capacitance and resistance have more complicated dimensions in eiq than in CGS.
In short, we have gained little except, possibly, a neutral system which would not offend anyone's planetary pride. And this is not very surprising, because we don't know that e, i, and q are the building blocks of the universe.
"Let us carve nature at the joints," said Francis Bacon, meaning that we should adopt definition; and make distinctions corresponding to real differences in the physical world. But nature's joints turn out to be rather elusive; in fact, it seems likely that nature is a seamless unity. We carve up the universe of phenomena because that's the only way our minds can deal with it. But it is sobering to think how many supposed fundamentals exist only in our own heads.
THE END